somerandomathblog: Pascal's Triangle

Pascal's triangle is a very interesting piece of mathematics named for French mathematician Blaise Pascal, although this triangle was being studied centuries before him in China, India, Persia, Germany, and Italy. The is formed as such: Start with one at the top, then place the numbers below in a triangle formation, and add numbers as they combine, so you place two ones underneath, then place two ones on the sides again, but this time in the center they will add up to two. This pattern continues all the way down the triangle, which is infinite.
Image result for pascal's triangle

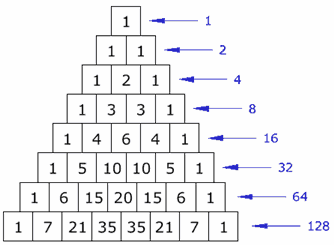
There are many patterns hidden within this triangle. For instance, if you write out the sums of the numbers in each row, you find the numbers double, starting with one, then two, then four, then eight, and so on. Also, if you look at the numbers formed by each row, you find that besides the top each are a multiple of eleven, This starts with 11(11 * 1), then 121(11*11), then 1331(11*121). This works all the way down the triangle, even with a row like 1, 5, 10, 10, 5, 1. Here, you carry over, resulting in the number 161,051(11 * 14641). This pattern is best seen with powers of 11, starting with 11⁰

(1), then going up 11¹(11), 11²(121), etc.
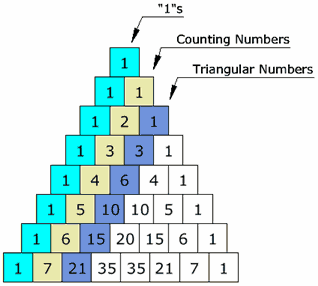
Image result for pascal's triangle doubles

Image result for pascal's triangle elevens

Another interesting thing is if you loom at the diagonals of this triangle. the sums of the numbers in the diagonals will give you the Fibonacci sequence. The diagonals in sequence also have things of interest, the first being ones, the second being counting numbers, the third being triangular numbers, and the fourth being square numbers.
Image result for pascal's triangle fibonacci sequence

Image result for pascal's triangle fibonacci sequence

Another strange and interesting thing one can see in this triangle are the Catalan Numbers. The Catalan Numbers are taking shapes and finding out how many triangles it can be divided into by connecting vertices. In Pascal's triangle these numbers are found by taking the even numbers in the center of almost every other row and subtracting the number adjacent to it.
Image result for catalan numbers

Image result for pascal's triangle catalan

Finally (but not really) If you highlight every even number in Pascal's Triangle, you get a fractal that is essentially Sierpinski's Triangle.
Image result for pascal's triangle